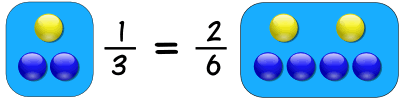Proportions
Proportion says that two ratios (or fractions) are equal.
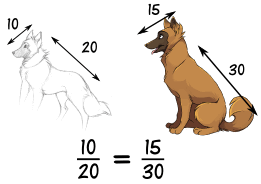
Sizes
When shapes are "in proportion" their relative sizes are the same.
Here we see that the ratios of head length to body length are the same in both drawings.
So they are proportional.
Making the head too long or short would look bad!
|  |
| ||||||||
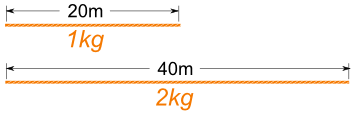
For example, let us say that the distance you travel is proportional to the time. This means that if you travel twice as long, you will go twice as far. If you travel three times as long, you will go three times as far. While if you travel half as long, you will go half as far.
By whatever ratio the time changes, the distance will change proportionally, that is, in the same ratio.
| ||||||||
| ||||||||
To begin with, we can only form ratios between things of the same kind: length to length, time to time, dollars to dollars, and so on. A ratio between things of different kinds ("This amount of money is half of this amount of time") makes no sense.
When we relate things of different kinds, as in "dollars per (for each) hour," that is not called a ratio but arate.
Example 1. In 4 hours, you can travel 110 miles. How far can you travel in 8 hours?
Answer. Since you travel twice as many hours -- 8 hours are twice as many as 4 hours -- then you will travel twice as many miles.
2 × 110 miles = 220 miles.
Proportionally, upon forming ratios between things of the same kind:
4 hours are to 8 hours as 110 miles are to 220 miles.
Example 2. Maria can earn $70 in 6 hours. How much will she earn in 18 hours? How much in 3 hours? How much in 27 hours?
Solution. Earnings are directly proportional to time. If Maria worksthree times as long -- 18 hours -- then she will earn three times as much. She will earn 3 × $70 = $210.
As for 3 hours, they are half of 6 hours: therefore she will earn halfof $70; she will earn $35.
Finally, 27 hours. Every 6 hours she earns $70. How many 6 hours are there in 27?
27 hours = 24 hours + 3 hours.
In 24 hours there are four 6's. She will earn 4 × $70 = $280.
In 3 hours, we have seen that she will earn $35.
Therefore, in 27 hours she will earn $280 + $35 = $315.
Equivalently, 27 hours are four and a half times6 hours. Therefore she will earn four and a half times $70.
When two quantities are directly proportional, we say that one of them varies directly as the other. In this example, wages vary directly as time.
Example 3. Which is a better value: 12 ounces for $5.00, or 48 ounces for $22.00?
Answer. 48 ounces are four times 12 ounces. Therefore we should expect the price to be four times more. But four times $5 is $20 -- which is less than $22. Therefore 12 ounces for $5 is a better value.
Example 4. Which is a better value: 15 ounces for $9.00, or 20 ounces for $11.00?
Answer. 20 ounces are 5 ounces more than 15 ounces. That is, 20 ounces are one third more. Now, one third more than $9.00 is
$9.00 + $3.00 = $12.00
Therefore, 20 ounces for $11.00 is a better value. You save $1.00.
Inverse proportionality
| ||||||||
| ||||||||
This means that if one of the quantities doubles, then the other will become half as large. For the inverse of the ratio 2 to 1 ("doubles") is the ratio 1 to 2 ("half"). The terms are exchanged.
Example 5. Let us suppose that the time it takes to do a job is inversely proportional to the number of workers. The more workers, the shorter the time.
Specifically: If 6 workers can do a job in 4 days, then how long will it take 12 workers?
Answer. The number of workers has doubled , going from 6 to 12. Therefore it will take only half as many days. It will take only 2 days.
Example 6. The speed that a car can achieve in 10 seconds is inversely proportional to its weight. (That is, the more the car weighs, the slowerit will be going.)
After 10 seconds, a car that weighs 2400 pounds can achieve a speed of 44 miles per hour. If the car weighed 1600 pounds, how fast would it be going?
Answer. What ratio has the new weight to the original weight -- 1600 pounds to 2400 pounds? 1600 is two thirds of 2400:
1600 is to 2400 as 16 is to 24 as 2 is to 3.
| ||||||||